Calculus III

(Math 2401, sections J1 & J2)

Mihalis Kolountzakis
School of Mathematics
Georgia Institute of Technology
686 Cherry Street NW
Atlanta, GA 30332

E-mail: kolount AT gmail.com

January 2007


Contents

1 What the course is about

Look here for the syllabus and more information.

You can also have a look at a previous semester's (2005) web site of mine for the same course.

2 Schedule

Main lectures: MWF 14:05 - 14:55, in Skiles 202.

My office hours are: Thu 10-12 in my office (Skiles 133).

You're also welcome to ask me questions any time you see me, anywhere.

Recitation sessions: TTh 14:05-14:55 in Skiles 202 (instructor Daniel Tiefa)

Dan's office hours are TBA.

3 Grading

There will be quizzes each Thursday (except for the first one) for the last 15 minutes of the session. One problem will be asked among those (or very much resembling one of those) that were assigned as homework.

The quizzes are worth 20% of the grade (the two smallest quiz scores will be discounted for each of you), each of the two midterms (Feb 9 and March 14) is worth another 20% and the final is worth 40%.

4 Course Progress

4.1 M, 1/8/07: Vector valued functions of a real variable

We covered §13.1. We saw examples of functions of a real variable whose values are 2D or 3D vectors. We defined the limit

\begin{displaymath} \lim_{t \to t_0} \vec f(t), \end{displaymath}

and we saw that the limit can be computed by evaluating the limits of the coordinate functions. We also defined the derivative and the integral of such a function and spoke briefly about how to draw the curve that such a function defines.

HW problems: §13.1: 1, 2, 15, 16, 21, 22, 27, 28, 39, 41.

4.1.1 ANNOUNCEMENT: Office hours change for first week

For the first week only, my office hours will be held on Friday, 3-5.

4.2 W, 1/10/07: Differentiation rules for vector-valued functions

We saw how to compute the derivatives of vector valued functions which have been made by piecing together, in various ways, other functions. For example, we saw that if $u(t)$ is a scalar function and $\vec f(t)$ is a vector function then the derivative of the function $\vec F(t)$ which is defined by

\begin{displaymath} \vec F(t) = u(t) \vec f(t) \end{displaymath}

is given by

\begin{displaymath} \vec F'(t) = u'(t) \vec f(t) + u(t) \vec f'(t). \end{displaymath}

We saw various such rules and proved a few.

HW problems: §13.2: 1, 2, 4, 6, 7, 9, 11, 17, 18, 21, 22, 27, 29, 32.

4.2.1 ANNOUNCEMENT: Midterm exam dates

The first midterm exam will take place on Fri, Feb 9, during our regular meeting and in the same place.

The second midterm will take place on Wed, Mar 14, also during our regular meeting and in the same place.

4.3 F, 1/12/07: Parametrized curves and their tangents

We saw a few examples of vector-valued functions viewed as parametrizations of curves in space. We saw that when $\vec r(t)$ traverses a curve $C$ then $\vec r'(t)$ is a tangent vector of $C$ at point $t$, and solved some problems which asked geometric questions about curves that had been described in parametric form. We also saw how to find the intersection points of two curves that have been given to us in parametrized form. One must be careful in this problem not to use the same name of the parameter for both curves.

Some of the problems listed below you cannot solve without reading the rest of §13.3 (we will cover this on Wednesday).

HW problems: §13.3: 1-4, 9, 14, 15, 18, 35-38.

4.4 W, 1/17/07: Tangent and normal vector. Length of a curve.

We finished §13.3: talked about the unit tangent vector $\vec T(t)$ to a curve at point $\vec r(t)$ and the principal normal vector $\vec N(t)$ as well as the osculating plane they define. We computed an example.

Then we saw how to calculate the length of a curve parametrized by the vector function $\vec r(t)$, for $a \le t \le b$. We computed some examples and also saw by example that the formula we gave for arc-length does not depend on the parametrization of the curve. That is we took two different paraetrizations of a specific curve (a circle, actually) and used our length formula with both of these parametrizations and we got the same result, as we should.

HW problems: §13.4: 1-4, 8, 9, 17, 23.

4.4.1 ANNOUNCEMENT: Office hours tommorrow

My office hours tommorrow, Thursday Jan 18, will be held from 9-11 (not 10-12) as I want to go to a lecture at 11 (this change may become permanent).

If any of your planned to see me from 11-12, please send me a message to set up an appointment (but not on Thursday).

4.5 F, 1/19/07: Parametrization by arc-length. Curvature of plane curves.

We covered the part of §13.5 that concerns plane curves and their curvature. We saw how to reparametrize a curve which has been given to us in parametric form $\vec r(t)$ in such a way that the parameter is now $s$ the arc-length along the curve. This parametrization has the property, which characterizes it, that the velocity vector has constant magnitude equal to $1$. The curvature of a plane curve is defined as the derivative with respect to $s$ of the angle formed by the tangent line to the curve and the $x$-axis, in absolute value. (This definition will have to be amended next time in order to define the curvature of space curves.) We then saw formulas that allow us to calculate the curvature given the parametrization of the curve. We applied these formulas to several examples. Finally we defined the radius of curvature and the center of curvature.

Of the following problems, do now those that concern plane curves, and leave those about space curves for after having finished §13.5.

HW Problems: §13.5: 1, 2, 5, 6, 10, 13, 14, 21, 22, 41, 42, 58.

4.6 M, 1/22/07: Curvature of space curves. Decomposition of the acceleration vector.

We saw how one defines the curvature $\kappa$ of space curves (curves which are not known to be contained in a plane) and computed some examples.

We also saw that acceleration of a particle moving along a curve $\vec r(t)$, that is the vector

\begin{displaymath} \vec a(t) = \vec r''(t) \end{displaymath}

is a linear combination of the two vectors $\vec T(t)$ and $\vec N(t)$, i.e. there are numbers $a_T$ and $a_N$, such that

\begin{displaymath} \vec a(t) = a_T \vec T(t) + a_N \vec N(t). \end{displaymath}

We then saw how to computed these coefficients $a_T$ and $a_N$ and did so in some examples.

Do the problems of §13.5, listed above, which you could not do before today's lecture.

4.7 W, 1/24/07: Applications of vector calculus to Mechanics

We covered §13.6. We saw how to apply some of the things we've seen so far to problems of Mechanics (motion of particles under certain forces). In particular, we proved Kepler's 2nd law which states that if a particle is moving under the influence of central force (force parallel to the location vector) then its location vector sweeps out equal areas in equal times.

We did not have time to cover the subsection on initial value problems. Please go over Examples 3 and 4 on p. 809 and 810.

HW Problems: §13.6: 1-4, 8, 9, 11, 14, 15.

4.8 F, 1/26/07: Initial Value Problems. Scalar functions of several variables.

We talked a little about how to solve initial value problems of the second order such as those that arise from Newton's equation in kinematics (read §13.6).

Then we moved on to §14.1 and talked about real-valued functions of two or three variables

\begin{displaymath} f(x, y), f(x, y, z). \end{displaymath}

We talked about the domain of a function (for which pairs $(x,y)$ or triples $(x,y,z)$ is the function defined). We gave several examples of functions defined by formulas and sought to find the domain of these functions by defining them on the largest set of points where the formulas make sense.

HW Problems: §14.1: 1-10, 35, 37, 39.

4.9 M, 1/29/07: Quadric surfaces

We first remembered what kind of curves in the plane have an equation $f(x,y)=0$ which is a polynomial in $x$ and $y$ of degree at most $2$, a quadratic equation. These are the so-called conic sections: ellipse, hyperbola, parabola, straight line, a pair of straight lines or a single point. Next we wrote down the general quadratic equation in three variables $x, y, z$, that is an arbitrary polynomial in these variables whose degree is at most $2$. The case now are many more and I am not asking that you memorize the entire list in your book (§14.2) but that you learn, as we did in class, how to figure out the shape of a given surface, whose equation you're given, by cleverly fixing some of the variable to appropriate values (in your book these are called intercepts and traces), and also by taking advantage of the symmetries of the equation. Do learn the few examples we did in class.

HW Problems: §14.2: 2, 4, 10, 12, 20, 22, 26, 40, 43.

4.9.1 ANNOUNCEMENT: Note taker needed

A student note taker is needed in this course to take notes for a student with a disability. The note taker will be paid a stipend for this assignment. Skills needed are the ability to take accurate, legible, and organized notes and a commitment to attend every lecture. If interested, please contact Tina Allen via office phone at 404-894-2563 or via email at notetaker@vpss.gatech.edu as soon as possible. Be sure to indicate the Professor's name, time, day and course number/ section in the subject line of the announcement.

4.10 W, 1/31/07: Level curves and surfaces; Partial derivatives of functions of many variables

We saw how to present geometric information about a function $f(x,y)$ or $f(x,y,z)$ (or its graph, representable by the equation $z=f(x,y)$ or $u=f(x,y,z)$) by drawing its level curves for two-variable functions, i.e. the curves whose equation is given by $\ell = f(x,y)$ for various values of $\ell$, or its level surfaces (three-variable case) which are the surfaces representable by the equation $\ell = f(x,y,z)$, again for various values of $\ell$.

HW Problems: §14.3: 4, 5, 8, 14, 19, 20, 21, 25, 28.

Next we saw how one defines the partial derivatives (with respect to each of the variables) of functions of more than one variable, and saw how to compute some examples by treating all variables but the one we're differentiating with as constants.

4.10.1 ANNOUNCEMENT: Office hours

My apologies to those of you (if any) who came to my office hours Thursday. I entirely forgot.

I will be in my office from 4-5 tomorrow Friday.

Mihalis

4.11 F, 2/2/07: Partial derivatives. Open sets.

We finished §14.4, by giving more examples of how to compute the partial derivatives of functions of several variables and by talking about the geometric significance of the partial derivatives of a function $f(x,y)$.

HW Problems: §14.4: 1-10, 23, 41, 53.

We then started speaking about §14.5. We defined the $\delta$-neighborhood (and deleted neighborhood) of a point $\vec x \in {\mathbf R}^\nu$, where $\nu = 1,2,3$. We also defined the concept of an open set $O \subseteq {\mathbf R}^\nu$ and saw, as an example, that the upper half plane $H \subseteq {\mathbf R}^2$:

\begin{displaymath} H = {\left\{{(x,y): y>0}\right\}} \end{displaymath}

is indeed an open set.

4.11.1 ANNOUNCEMENT: Practice exam

You can find a practice exam (for 50 min) here in PDF.

4.12 M, 2/5/07: Open and closed sets, interior and boundary points

We defined what closed sets are (the complements of open) sets as well as what the interior points of a set are and its boundary points. We saw several examples, both in dimension 2 as well as in dimension 1.

HW Problems: §14.5: 1-20.

4.12.1 ANNOUNCEMENT: First Test

For the first test (this Friday, in class) you are expected to know all that has been taught to you by today (Monday). Please come early so as to start on 14:05 sharp.

There will be a quiz on Thursday as usual.

4.13 W, 2/7/07: Review

We went over the practice exam and aswered some questions.

4.14 F, 2/9/07: First Test

We had our first test. Here are the problems.

  1. A particle of mass $m=10$ moves according to the law $\vec r(t) = (t, t^2, t^3)$. Find, as a function of $t$, its velocity, its speed and the force which is applied on the particle.

    Solution: Velocity: $\vec v(t) = \vec r'(t) = (1, 2t, 3t^2)$.
    Speed: $v(t) = \frac{ds}{dt} = {\left\Vert{\vec v(t)}\right\Vert} = \sqrt{1+4t^2+9t^4}$.
    Force: $\vec F(t) = m \vec r''(t) = 10(0, 2, 6t) = (0, 20, 60t)$.

  2. For the curve parametrized by $\vec r(t) = (\cos t, \sin t, e^t)$, $t \ge 0$, find
    (a) the velocity vector $\vec v(t)$, and
    (b) a parametrization of the tangent line to the curve at the earliest (smallest $t$) moment that line becomes parallel to the $xz$-plane.

    Solution: (a) $\vec v(t) = \vec r'(t) = (-\sin t, \cos t, e^t)$.
    (b) For a vector to be parallel to the $xz$-plane means that its $y$-coordinate is $0$. This happens for the first time at $t=\pi/2$. At that moment the velocity vector is $\vec v(\pi/2) = (-1,0,e^{\pi/2})$. A parametrization of the tangent line (which goes through the point $\vec r(\pi/2) = (0,1,e^{\pi/2})$ and has the direction of the vector $\vec v(\pi/2)$ is

    \begin{displaymath} \vec R(t) = \vec r(\pi/2) + t \vec v(\pi/2) = (0,1,e^{\pi/2}) + t(-1,0,e^{\pi/2}) = (-t, 1, e^{\pi/2}(1+t)). \end{displaymath}

  3. Let $f(x,y,z) = \ln(x^2+y^2+z^2 -4)$.
    (a) Find the maximum domain where $f$ is defined and describe it geometrically.
    (b) Compute all three partial derivatives of $f$.

    Solution: (a) The argument of the function $\ln(\cdot)$ must be positive, hence the domain is

    \begin{displaymath} D = \{(x,y,z): x^2+y^2+z^2 > 4\}. \end{displaymath}

    This is the outside of the sphere centered at the origin with radius 2, without the surface of the sphere.
    (b) $f_x = \frac{2x}{x^2+y^2+z^2-4}$, $f_y = \frac{2y}{x^2+y^2+z^2-4}$, $f_z = \frac{2z}{x^2+y^2+z^2-4}$.

  4. (a) Describe in geometric language the region of the plane

    \begin{displaymath} A = \{(x,y): xy \ge 0\}. \end{displaymath}

    Find its interior and boundary points.
    (b) Same question for the region

    \begin{displaymath} B = \{(x,y): xy>0\}. \end{displaymath}

    Solution: (a) This is the region where $x$ and $y$ have the same sign or some of them is zero. This is the first and third quadrant of the plane, together with the $x$- and $y$-axes.

    Any point of that set which is not on the axes is an interior point as one can draw a disk centered at it which is entirely in the set. Any point on the axes is not an interior point as any disk around such a point will contain points outside the first and third quadrant.

    For this reason all points of the axes are boundary points. There are no other boundary points as any point inside a quadrant and not on an axis will have a small disk around it which contains either only points of the set or of its complement and not from both.
    (b) The only difference of this shape from that of (a) is that the $x$- and $y$-axes are not part of the set now. However the set of interior points and the set of the boundary points remains the same as that of (a). (In this case the set of boundary points, namely the two axes, is not part of the set itself.)

4.15 M, 2/12/07: Continuity of multivariable functions, partial derivatives and mixed second order derivatives

We defined formally what the limit of a two-variable function is when $(x,y) \to (x_0, y_0)$, and when a function is continuous at a point $(x_0,y_0)$. We calculated the limits using the definition for some very simple functions.

We also saw examples of functions of two variables which exhibit strange behaviour, by one variable standards. For example we saw a function which is everywhere continuous with respect to each of the variables and has everywhere partial derivatives, yet is not continuous at (0,0).

HW Problems: §14.6: 1-5, 21, 23, 24, 26, 27.

4.15.1 ANNOUNCEMENT: First test grades

You can find your grade here in PDF.

4.16 W, 2/14/07: Differentiability of multivariable functions. The gradient of a function

We defined what it means for a function of many variables to be differentiable at a point $\vec x$,and also defined the gradient of a differentiable function $f$ at $\vec x$, as the only vector $\vec L$ that makes the following true:

\begin{displaymath} f(\vec x + \vec h) - f(\vec x) = \vec L \cdot \vec h + o\left({\left\Vert{\vec h}\right\Vert}\right). \end{displaymath}

We then saw that if a function has continuous partial derivatives at a point then it is differentiable there and the components of its gradient are just its partial derivatives with respect to the corresponding variables.

See, for example, this page for the big-O and little-o notation that we talked about today.

HW Problems §15.1: 12-16, 33-37, 39, 40.

4.17 F, 2/16/07: Gradients and directional derivatives

We talked about the concept of directional derivatives and how to compute them using the gradient of a function. Also saw that the direction of the gradient is the direction of maximum rate of increase of a function. Saw several examples.

HW Problems §15.2: 11-14, 23-26, 40, 41.

4.18 M, 2/19/07: Mean Value Theorem in the multivariable case; Chain rules

We went over the Mean Value Theorem for scalar functions of one variable, and used it to prove the mean value theorem for scalar functions of a vector variable. We remarked that the Mean Value Theorem is not true for vector valued functions. We saw some consequences of the MVT: if two functions have the same gradient in a connected open set then they differ by a constant.

Next we reviewed the chain rule for functions of one variable and saw the form it takes for the composition of a scalar function of a vector variable with a vector valued function of one variable. We saw how to construct the dependency diagram when we have many quantities that depend on others, and use it to derive the appropriate chain rule.

Please read from §15.3 about implicit differentiation.

HW Problems §15.3: 1, 3, 4, 6-8, 17, 18, 25, 27, 29, 30, 36, 58.

4.19 W, 2/21/07: The gradient as normal vector to level curves and surfaces

We pointed out that $\nabla f(\vec r)$ is a normal vector to the curve (or surface) $f(\vec r) = C$, $C$ a constant. We used this to compute normal and tangent vectors to curves and surfaces amd also angles between curves and surfaces. These angles are defined to be the corresponding angles between their tangent objects at the intersection. For example, if we are seeking the angle between a curve and a surface which intersect at a point $\vec P$ we must measure the angle between a tangent vector of the curve at $\vec P$ and the tangent plane to the surface at $\vec P$. This is most easily measured by first finding the angle between the tangent to the curve and the normal to the tangent plane, and then subtracting that angle from $\pi/2$.

HW Problems §15.4: 1, 2, 10, 11, 19, 20, 26, 27, 28, 34, 36.

4.20 F, 2/23/07: Local extrema

We saw what is the analogue in two variables of the criteria, involving first and second derivatives, that we know for deciding where a function's local maxima and minima are. The first stage of the method is to locate the points where the function's gradient vanishes. Each of those points is then checked using higher order partial derivatives of the function at that point in order to decide if there is a local extremum at that point or if it is a saddle point.

HW Problems §15.5: 1, 2, 5-8, 25, 26.

4.21 M, 2/26/07: Absolute extrema

We saw how to find the (global) minima or maxima of a function of two variables defined in a closed and bounded domain. We have to find the critical points in the interior of the domain and then parametrize our boundary, which gives rise to a function of one variable corresponding to our function being evaluated on the boundary. This one-variable function we extremize (find its extrema) separately. The extrema of this one-variable function along with the critical points in the interior are then compared in the value of the function at them to find the global extrema.

HW Problems §15.6: 1-6, 19-22, 27.

4.22 W, 2/28/07: Function extremization under side conditions

We talked about the problem of finding the minimum or maximum of a function $f(\vec x)$ when $\vec x$ is not free to take any values in the domain of definition of $f$ but it has to satisfy some condition, which is usually given in the form $g(\vec x) = 0$. Sometimes one can solve $g(\vec x)$ for one of the variables in $\vec x$ and substitute the resulting expression in $f(\vec x)$ thus getting a problem of ordinary function extremization without side conditions and in one variable less. We saw two such examples but this method is most often inapplicable as it is not easy to solve $g(\vec x) = 0$ for one of the variables,especially if $\vec x$ is a three-component vector and $g$ is non-linear. Even if possible the resulting expression for $f$ may be too complicated to work with.

We then saw the method of Lagrange (or Lagrange multipliers as it is commonly known), which allows one to solve the above problem by solving a, generally non-linear, system of equations in the unknowns $\vec x$ and $\lambda$, the latter being a auxiliary variable which is not used except to help find the values of $\vec x$. The system of equations is

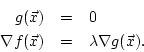
\begin{eqnarray*} g(\vec x) & = & 0\\ \nabla f (\vec x) & = & \lambda \nabla g(\vec x). \end{eqnarray*}


The last equation is actually many, as many as the number of components in $\vec x$ (two or three in our cases).

HW Problems §15.7: 1-4, 13, 15, 18, 19, 21, 23.

4.23 F, 3/2/07: Function extremization under side conditions, continued

We gave some more examples of the method of Lagrange multipliers and saw how it applies to the case of a function of three variable subject to two conditions.

We also saw that there is a way to avoid introducing the Lagrange multiplier (the $\lambda$ variable, which we throw away after solving the system of equations). This can be done by checking the parallelism between the two fectors $\vec{\nabla}f$ and $\vec{\nabla} g$ (the gradients of the function to be optimized and the constraint function) by checking the equation

\begin{displaymath} \vec\nabla f \times \vec\nabla g = \vec 0. \end{displaymath} (1)

That is, our system of equations consists now of the above equation plus the constraint $g(x,y,z) = 0$. It appears odd however that we have three variables ($x$, $y$ and $z$) but four equations (the equation (1) is three equations written as one, as it is an equation between vectors with three components). So how can we expect this system to be generally solvable?

The answer is that the vector equation $\vec A \times \vec B = \vec 0$ is really only two equations. If one writes it out (using the determinant form) one notices immediately that any of the resulting three equations can be obtained from the other two, so one can throw away any one of them (but only one) without changing the solutions to our system. Now it is clear that we have three equations in three unknowns.

4.24 M, 3/5/07: Summation sign

We saw how to use the summation sign and iterated (multiple) summation sign via several examples. We also evaluated several simple sums.

HW Problems §16.1: 1-4, 13-17.

4.24.1 ANNOUNCEMENT: Practice exam

You can find a practice exam (for the 50 min 2nd midterm) here in PDF.

4.24.2 ANNOUNCEMENT: Note taker needed

Note Taker Announcement:

A student note taker is needed in this course to take notes for a student with a disability. The note taker will be paid a stipend for this assignment. Skills needed are the ability to take accurate, legible, and organized notes and a commitment to attend every lecture. If interested, please contact Christina Bibbs at notetaker@vpss.gatech.edu, and be sure to indicate the Professors name, time, day and course number in the subject line of your email. Thank you so much for your willingness to assist our office in this process. Please contact our office if we can be of any assistance.

4.25 W, 3/7/07: Integral of a continuous function of two variables

We defined the integral of a function $f(x,y)$ over a rectangle $R$ via lower and upper sums corresponding to partitions of $R$. We evaluated, using the definition, only some simple integrals.

HW Problems §16.2: 1, 2, 6, 7, 10, 11.

4.26 F, 3/9/07: Double integrals by repeated integration

We stated the Mean Value Theorem for double integrals over connected domains (§16.2).

When a domain is such that all its intersections with lines parallel to the $y$-axis are intervals, and the set of $x$-values used in the domain consititute an interval the domain is called of Type I (and of Type II if the same properties hold with $x$ and $y$ reversed). For such a domain we saw how to evaluate a double integral of a function as a single integral whose function to be integrated is an integral itself.

HW Pproblems §16.3: 1-6, 13, 14, 33, 34, 43, 46.

4.27 M, 3/12/07: Review session

We did not cover any new material. Instead we went over the practice exam, in preparation for Wednesday's exam.

4.28 W, 3/14/07: Second Test

We had our second test. Here are the problems:

  1. A rectangular box is inscribed in the sphere $x^2+y^2+z^2 = a^2$ with its center at the origin and its sides parallel to the coordinate axes. (The corners of the box are all on that sphere.) Find the maximum volume of such a box.

    Solution: Suppose the vertex of the box lying in the first octant has coordinates $(x,y,z)$, with $x, y, z>0$. It follows that the volume of the box is

    \begin{displaymath} V(x,y,z) = (2x)\cdot(2y)\cdot(2z) = 8xyz. \end{displaymath}

    We have therefore to maximize the function $V$ subject to the condition
    \begin{displaymath} g(x,y,z) = x^2+y^2+z^2-a^2=0. \end{displaymath} (2)

    We easily calculate

    \begin{displaymath} \vec\nabla V=(8yz, 8xz, 8xy), \vec\nabla g = (2x, 2y, 2z). \end{displaymath}

    Following the method of Lagrange multipliers we have to solve the system (in $x, y, z, \lambda$)

    \begin{displaymath} \vec\nabla V = \lambda \vec\nabla g, g=0. \end{displaymath}

    Expanding it we get the four equations

    \begin{displaymath} 8yz = \lambda 2x, 8xz = \lambda 2y, 8xy = \lambda 2z, x^2+y^2+z^2 = a^2. \end{displaymath}

    Since $x, y, z>0$ we can divide the first and second equations to get $x=y$. Similary we get $y=z$ and the last equation now gives $x=y=z=a/\sqrt{3}$ (the box is a cube). It follows that the maximum volume is

    \begin{displaymath} V(a/\sqrt{3}, a/\sqrt{3}, a/\sqrt{3}) = 8\cdot 3^{-3/2} a^3. \end{displaymath}

  2. Find the absolute minimum and maximum of the function $f(x,y)=x^2+4y^2+x-2y$ in the closed region bounded by the ellipse $\frac{x^2}{4}+y^2=1$.

    Solution: We have

    \begin{displaymath} \vec\nabla f(x,y)=(2x+1, 8y-2) \end{displaymath}

    and setting $\vec\nabla f = 0$ we get the single solution

    \begin{displaymath} (x_0, y_0) = (-1/2, 1/4) \mbox{with} f(x_0,y_0)=-1/2. \end{displaymath}

    This critical point of $f$ is inside the ellipse as it satisfies

    \begin{displaymath} x_0^2/4 + y_0^2 = 1/4 \le 1. \end{displaymath}

    We now turn our attention to the boundary of the region, namely on the ellipse itself, which can be parametrized as

    \begin{displaymath} \vec r(t) = (2\cos t, \sin t), 0\le t \le 2\pi. \end{displaymath}

    We thus have to find the maximum of the one-variable function

    \begin{displaymath} g(t) = f(\vec r(t)) = 4 \cos^2 t + 4\sin^2 t +2\cos t - 2\sin t = 4+2\cos t - 2\sin t. \end{displaymath}

    Differentiating $g$ with respect to $t$ we get

    \begin{displaymath} g'(t) = -2(\sin t + \cos t), \end{displaymath}

    which vanishes precisely when $\cos t = -\sin t$, that is for $t=3\pi/4$ and $t=7\pi/4$. These two values of $t$ correspond to the two points

    \begin{displaymath} (-2/\sqrt{2}, 1/\sqrt{2}), (2/\sqrt{2}, 1/\sqrt{2}) \end{displaymath}

    on the ellipse, on which the function $f$ takes the (positive) values

    \begin{displaymath} 4-2\sqrt{2} \mbox{and} 4+2\sqrt{2}. \end{displaymath}

    It follows that $\min f = -1/2$ (the value at the critical point inside the ellipse) and $\max f = 4+2\sqrt{2}$ (the largest of the two values we found on the boundary).
  3. Find the points on the surface $x+y+z+xy-x^2-y^2=0$ at which the tangent plane to the surface is horizontal. Write the equations of the corresponding tangent planes.

    Solution: The surface is a level surface of the function

    \begin{displaymath} f(x,y,z) = x+y+z+xy-x^2-y^2, \end{displaymath}

    therefore the normal vector to the surface at point $(x,y,z)$ is just

    \begin{displaymath} \vec\nabla f(x,y,z) = (1+y-2x, 1+x-2y, 1) \end{displaymath}

    and this vector must have its first two coordinates equal to $0$ in order for the tangent plane to the surface at $(x,y,z)$, which has that vector as normal vector, to be horizontal. This gives rise to the system in $x, y, z$:

    \begin{displaymath} 2x - y =1, 2y - x = 1, x+y+z+xy-x^2-y^2 = 0, \end{displaymath}

    which we easily solve (start by subtracting the second equation from the first) to get

    \begin{displaymath} x = 1, y = 1, z = -1 \end{displaymath}

    as its only solution. The equation of the (horizontal) tangent plane at that point is simply $z= -1$.
  4. Let $u=u(x,y)$ be a function of $x$ and $y$. Let also $x=r \cos\theta$, $y=r\sin\theta$. Calculate the second order partial derivative $u_{rr}$ in terms of: $r$, $\theta$ and the partial derivatives of $u$ with respect to the variables $x$ and $y$.

    Your formula for $u_{rr}$ may not involve partial derivatives of $u$ with respect to $r$ or $\theta$.

    Solution: We have $x_r = \cos \theta$ and $y_r = \sin\theta$. By the chain rule we get

    \begin{displaymath} u_r = u_x x_r + u_y y_r = u_x \cos\theta + u_y \sin\theta. \end{displaymath}

    We now differentiate this equation with respect to $r$ to get

    \begin{displaymath} u_{rr} = \cos\theta (u_x)_r + \sin\theta (u_y)_r. \end{displaymath}

    To get $(u_x)_r$ and $(u_y)_r$ we apply the chain rule again to get

    \begin{displaymath} (u_x)_r = u_{xx} \cos\theta + u_{xy}\sin\theta, (u_y)_r = u_{xy}\cos\theta + u_{yy}\sin\theta. \end{displaymath}

    Putting this together we get

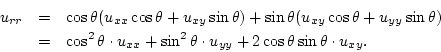
    \begin{eqnarray*} u_{rr} &=& \cos\theta (u_{xx} \cos\theta + u_{xy}\sin\theta) +... ...\sin^2\theta\cdot u_{yy} + 2 \cos\theta \sin\theta \cdot u_{xy}. \end{eqnarray*}


4.28.1 F, 3/16/07: ANNOUNCEMENT: Test results

You may find them here in PDF.

4.29 F, 3/16/07: Test problems

We went through the problems that were on the last test and their solutions.

4.30 M, 3/26/07: Double integrals in polar coordinates

We saw how to evaluate a double integral using polar coordinates. The first task is to find the domain $\Gamma$ in the $(r,\theta)$-plane which corresponds to the given domain $\Omega$ (in the $(x,y)$-plane). For example, if $\Omega$ is the unit disk in the cartesian plane (the $(x,y)$-plane) then $\Gamma$ is a rectangle in the $(r,\theta)$-plane defined by

\begin{displaymath} 0 \le r \le 1, 0\le \theta < 2\pi. \end{displaymath}

Next we transcribe the function to be integrated, from the cartesian variables into the polar variables. This is achieved by just substituting $r\cos\theta$ for $x$ and $r\sin\theta$ for $y$. Last, we replace the ``area element'' $dx dy$ by the expression $rdr d\theta$.

Please read by yourselves the evaluation of $\int_{-\infty}^\infty e^{-x^2} dx = \sqrt\pi$ from the end of §16.4. This is a very important integral in all mathematics and especially its applications to science and engineering.

HW Problems §16.4: 1, 2, 5, 6, 9, 10, 17-20, 23, 24.

4.31 W, 3/28/05: Applications of double integrals to mechanics

We covered a couple more examples from §16.4 and proved the identity

\begin{displaymath} \int_{-\infty}^\infty e^{-x^2} dx = \sqrt\pi \end{displaymath}

using polar integration.

We covered some of the examples in §16.5. We saw how to compute the mass of a two-dimensional domain (a ``plate'') with variable density, and also how to compute its center of mass. We also saw the definition of the moment of inertia of a plate (with variable density) rotating around a line or point. We evaluated some relevant double integrals.

HW Problems §16.5: 1-4, 11, 12, 14, 17, 25.

4.32 F, 3/30/07: Triple integrals

We saw briefly how triple integrals are defined (in a completely analogous way to double integrals, so we did not insist much on §16.6) and proceeded to evaluate some triple integrals by repeated integration.

HW Problems §16.7: 3-6, 11, 14-16, 21-22.

4.33 M, 4/2/07: Triple integrals in cylindrical coordinates

We showed how to compute a triple integral in cylindrical coordinates.

HW Problems §16.8: 1-8, 11, 12, 17, 18, 25, 26.

4.34 W, 4/4/07: Spherical coordinates for triple integrals

We introduced the spherical coordinate system and how to use it for evaluation of triple integrals. We saw several examples of how to transform the domain of integration from cartesian to spherical coordinates and carry out the integration in spherical coordinates (the form $dx dy dz$ becomes now $\rho^2 \sin\phi d\rho d\phi d\theta$).

HW Problems §16.9: 1-4, 9-14, 16, 19, 20, 24, 26, 27.

4.35 F, 4/6/07: General change of variables in multiple integrals

We saw the general procedure for evaluating a multiple (double or triple) integral over a domain $\Omega$ after first doing a change of variables. This is essentially a way of parametrizing $\Omega$ using two or three variables (depending on the whether the domain $\Omega$ is in the plane or space) which run over a more convenient domain $\Gamma$. We say how to do this when $\Omega$ is a parallelogram (and we got a parametrization with paramaters $u$ and $v$ running through the rectangle $0 \le u,v \le 1$) and also in some other cases. We also saw that the form $dx dy$ transforms into the form ${\left\vert{J(u,v)}\right\vert} du dv$ (and similarly in three dimensions), where $J(u,v)$ is the so-called Jacobian determinant, which can be computed from the functions $x=x(u,v)$ and $y=y(u,v)$.

HW Problems §16.10: 1-2, 8-10, 12-14, 19, 20, 27, 29.

4.36 M, 4/9/07: Line integrals

We defined the line integrals of a vector field $\vec f = \vec f (x,y,z)$ along a curve $C$ given parametrically by $\vec r(t)$, $a \le t \le b$, as the expression

\begin{displaymath} \int_C \vec f \cdot d\vec r = \int_a^b \vec f(\vec r(t))\cdot \vec r'(t) dt. \end{displaymath}

We proved that the line integral so defined is independent of which parametrization is being used for the curve $C$, as long as we do not change the orientation. We computed some examples.

HW Problems §17.1: 1-4, 7, 15, 16, 20, 21, 23, 25, 28-30.

4.37 W, 4/11/07: The fundamental theorem of calculus for line integrals

This says that if a vector field $\vec F$ is a gradient field, then a line integral of that along a curve $C$ equals the value of $f$ (where $\nabla f = \vec F$) equals $f(\vec b) - f(\vec a)$ where $\vec a$ and $\vec b$ are the endpoints of $C$. This is true in two and three dimensions, and, in two dimensions, in order to decide if $\vec F=(P,Q)$ is a gradient field we need to verify that $P_y = Q_x$ when the domain $\Omega$, where $\vec F$ is defined, is simply connected (i.e. connected and with no ``holes'').

HW Problems §17.2: 1-4, 12, 13, 22, 24-26, 28.

4.37.1 W, 4/11/07: ANNOUNCEMENT: Office hours tomorrow

My office hours tomorrow, April 12, will last only until 11am.

4.38 F, 4/13/07: Work and conservation of energy

We discussed kinetic energy and why its change is due to the work is done by the force field on the particle. Also we talked about conservative (gradient) fields and the potential function.

HW Problems §17.3: 1-3, 6, 7, 8.

4.39 M, 4/16/07: New way of writing line integrals; integrals with respect to arc-length

We saw an alternative way of writing the line integral $\int_C \vec{h}\cdot d\vec r$ as $\int_C Pdx + Qdy + Rdz$, where $\vec h = (P,Q,R)$. We also saw the line integral w.r.t. arc-length, denoted by $\int_C f ds$, where $f$ is a scalar function.

HW Problems §17.4: 2, 3, 5, 17, 18, 26, 27, 29, 30, 32, 36.

4.40 W, 4/18/07: Green's theorem

We stated Green's theorem. This expresses a line integral along a closed curve as a double integral over the interior of that curve. We proved this in the case when the domain if of Type I and of Type II and showed how one proves this if the domain is more general by cutting up the domain into a finite number of non-overlapping parts each of which is of both Type I and II. We also explained how to parametrize the boundary of a domain if that is not simply connected or even not connected (walk along the boundary in such a way that the domain is always on your left).

HW Problems §17.5: 1, 2, 5, 6, 18-20, 26, 28, 30, 31.

4.41 F, 4/20/07: Applications of Green's formula to evaluation of some double integrals

We saw how to apply Green's formula to derive a formula for the area of a polygonal region that has been described to us by the coordinates of its vertices. We also saw how to find an analogous formula for the centroid of the polygon and for the volume of the solid that arises if we rotate a polygonal region (which is part of the right half plane $x \ge 0$) about the $y$-axis.

4.42 M, 4/23/07: Surface area

We saw how to calculate the area of a surface which has been given to us in parametric form.

HW Problems §17.6: 1, 2, 5, 6, 15, 16, 17, 22, 23, 35, 36.

4.42.1 W, 4/25/07: ANNOUNCEMENT: Practice final exam.

You may find it here in PDF.

4.43 W, 4/25/07: Review session

I answered various questions of the students.

4.44 F, 4/27/07: Review session

We went through the practice final exam that was distributed on Wednesday.

4.44.1 M, 4/30/07: ANNOUNCEMENT: Final exam.

It will take place in Skiles 202 (lecture room), on Friday May 4, from 11:30 to 14:20.

4.45 F, 5/4/07: Final Test

We had our final exam. Here are the problems:

  1. Let the surface $S_1$ be defined by the equation $z=x^2+y^2$ (a paraboloid) and the surface $S_2$ be defined by the equation $y=x^2+z^2$ (also a paraboloid). The intersection of the surfaces $S_1$ and $S_2$ is a curve $C$ and the point $\vec P = (0,1,1)$ belongs to $C$ as its coordinates satisfy the equations of both $S_1$ and $S_2$. Find a unit tangent vector to the curve $C$ at $\vec P$.

    Hint: Use the normal vectors to $S_1$ and $S_2$ at $\vec P$.

  2. Find the point of the surface $z=x^2+y^2$ which is closest to the point $(2,2,1)$.
  3. Let $R>0$ and $\Omega = \{(x,y): x^2+y^2 \le R^2, x,y \ge 0\}$ be a quarter-disk in the first quadrant. Using polar coordinates compute the double integral

    \begin{displaymath} \int\int_\Omega e^{-x^2-y^2} dx dy. \end{displaymath}

  4. Compute the line integral

    \begin{displaymath} \oint_C \frac{(x+y) dx - (x-y) dy}{x^2+y^2} \end{displaymath}

    where $C$ is the circle of radius $R$ centered at $(0,0)$ oriented counterclockwise.

    Hint: Do not use Green's theorem.

  5. A simple closed curve $C$ in the $xy$-plane encloses a domain $\Omega$. Show that the moment of inertia of $\Omega$ with respect to the $z$-axis is equal to the line integral

    \begin{displaymath} \frac{1}{3} \oint_C x^3 dy - y^3 dx. \end{displaymath}

  6. Compute the volume of the solid defined by the three inequalities

    \begin{displaymath} z \ge 0, z^2 \ge x^2+y^2 \mbox{and} x^2+y^2+z^2 \le R^2. \end{displaymath}

4.45.1 S, 5/5/07: ANNOUNCEMENT: Final results

You may find them here.

I will be in my office on Sunday May 6, from 6 to 7pm, in case somebody wants to see an exam paper. After that the grades will be finalized.

Mon May 7 20:15:18 EDT 2007: There had been an error in the software that computed your numerical scores, which had as an effect that the quizzes were not counted (and possibly other effects). This error has now been corrected (I hope) and your numerical grades are now correctly computed (please, check yours, and let me know if you believe there is a numerical error in your posted numerical grade).

Some letter grades have inevitably been changed as the landscape changed, most of them for the better I believe, but not all.

I will enter the corrected grades into the system tomorrow but the process is slow and the changes may not show up online for several days.

I apologize for this mistake. I wish one of you had checked the numbers concerning him/her but, amazingly, no one did (at least, no one notified me of the discrepancy). Neither did I until today when I realized the error.


Mihalis Kolountzakis 2007-05-07